Jul21

Divisibility Criteria for 3, 4, 9 and 11

2 Comments

In this post we are going to learn about the criteria of divisibility by 3, 4, 9 and 11.

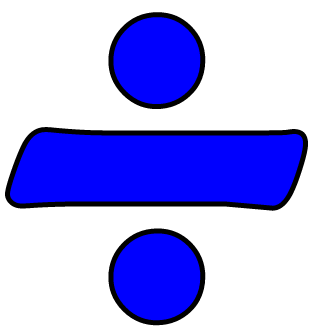
divisibility

Criteria of divisibility by 3

A number is divisible by 3 when the sum of its digits is a multiple of 3.

For example: Is 1098 divisible by 3?

We add all the digits of 1098:

1 + 0 + 9 + 8 = 18

1+ 8 = 9

9 is a multiple of 3, therefore 1098 is divisible by 3.

Criteria of divisibility by 4

A number is divisible by 4 when the last two numbers are divisible by 4.

Let’s look at an example. We want to know if 448 is divisible by 4, so we need to see if its last two numbers, 48, are divisible by 4.

48/4 = 12 and the remainder is 0.

Therefore, 448 is divisible by 4.

Criteria of divisibility by 9

A number is divisible by 9 when the sum of its digits is a multiple of 9.

For example, let’s check if 2610 is a multiple of 9.

2 + 6 + 1 + 0 = 9

Therefore 2610 is divisible by 9.

Criteria of divisibility by 11

A number is divisible by 11 when the sum of the numbers that occupy the even places minus the sum of the numbers that occupy the odd places is equal to 0 or a number which is a multiple of 11.

Is 5863 divisible by 11?

To find out if 5863 is divisible by 11, we identify which numbers are located in the even places and which numbers are located in the odd places.

Even places: 8 and 3.

We add them: 8 + 3 = 11

Odd places: 5 and 6.

We add them: 5 + 6 = 11

11-11 = 0

Therefore 5863 is divisible by 11.

Try Smartick for free to learn more math.

Learn More:

Fun is our brain’s favorite way of learning

Diane Ackerman

Smartick is a fun way to learn math

15 fun minutes a day
Adapts to your child’s level
Millions of students since 2009

Author
Recent Posts

Smartick

Content Creation Team.
A multidisciplinary and multicultural team made up of mathematicians, teachers, professors and other education professionals!
They strive to create the best math content possible.