Today we’re going to take a look at a very useful system of representing and working with monomials and polynomials: algebra tiles. They’re very intuitive and easy to use, and we’re currently incorporating them into our platform so that our students have this resource available when working with polynomials in their daily Smartick sessions.
If you’re not sure what a polynomial is, take a look at our previous entry about its properties before you continue.
Representing polynomials with algebra tiles
Algebra tiles are tiles of different colors and sizes. Each size corresponds to a degree of the different monomials of the polynomial. An example will make it easier to understand:
- This tile represents a unit. It corresponds to monomials with a degree of 0.
- This represents ‘x’. It corresponds to monomials with a degree of 1.
- Finally, this represents ‘x2‘. It corresponds to monomials with a degree of 2.
Therefore, if we want to represent the polynomial 2x2 + 4x + 5 it’s as easy as arranging the corresponding tiles:
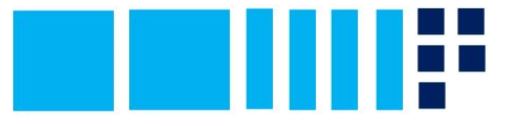
We’ve got 2 tiles of ‘x2‘, 4 tiles of ‘x’, and 5 tiles that represent a unit.
Easy, right?
Adding polynomials with algebra tiles
Adding polynomials is just as simple as representing them. In this case we’ll use polynomials with all positive monomials. We’ll take the polynomial that we represented before, 2x2 + 4x + 5, as the first summand. The second summand will be x2 + 2x + 3. Let’s represent them both with the algebra tiles:
Now we add the like tiles to find the result…
The polynomial result of the addition is 3x2 + 6x + 8.
It’s an easy and intuitive resource that helps us better understand polynomials and operations with them. You can make them yourself and practice with them anywhere you want!
If you want to know more about polynomials and other mathematical content, log in to Smartick and try it free.
Learn More:
- How to Subtract Polynomials with Help from Algebra Tiles
- Review the Properties of Polynomials
- Learn How to Add Polynomials with Examples
- Using Lego Blocks to Help with Addition of Fractions
- Using Mixed Numbers to Represent Improper Fractions
A multidisciplinary and multicultural team made up of mathematicians, teachers, professors and other education professionals!
They strive to create the best math content possible.
- The Language of Functions and Graphs - 07/01/2024
- Educational Technology: The Christodoulou Test - 05/06/2024
- Multiplication Activities in Smartick - 04/09/2024
Good