We are going to begin this post about adding fractions with three very simple questions:
1. If you had one apple and were given two apples, how many apples do you have now?
2. If you had one apple and were given half an apple, how many apples do you have now?
3. If you had half an apple and were given a fourth of an apple, how many apples do you have now?
Finished? If your answers match these we can continue:
- Now I have 3 apples.
- Now I have 1 and 1 half of an apple. Or, 3 halves of apples.
- Now I have 3 fourths of an apple.
I don’t know if you have done it before, but you have just added fractions with different denominators. In this post, we are going to see more exercises to learn how to do it and show you that it is much easier than you thought. Let’s go!
Adding Fractions with Different Denominators
In fact, you have been adding fractions with different denominators for some time. I’ll give you an example. Have you ever eaten a fourth of pizza and then had a little bit more – maybe an eighth of pizza? If you know how to calculate how much pizza you ate in this scenario it is because you have added fractions with different denominators.
However, if you don’t know how to do it, don’t worry! We are going to learn that now!
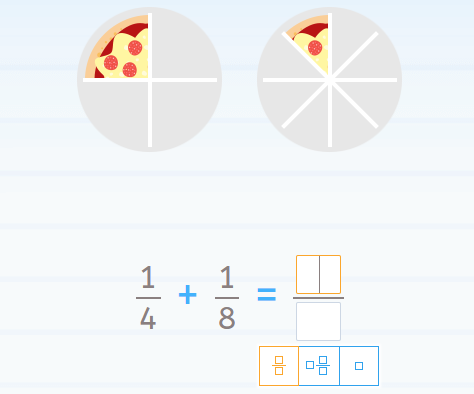
The image above represents this situation: a fourth of pizza plus an eighth of pizza.
If you look closely at the imageyou will see that the fourth is twice as big as the eighth. Therefore, saying that you have eaten one fourth is the same as saying that you have eaten two eighths.
If we think about this, adding the fractions 1 fourth + 1 eighth is the same as 2 eighths + 1 eighth.
Do you know how to solve the second addition problem?
If I eat two-eighths of a pizza at first and then eat an eighth of a pizza, I will have eaten three-eighths of a pizza.
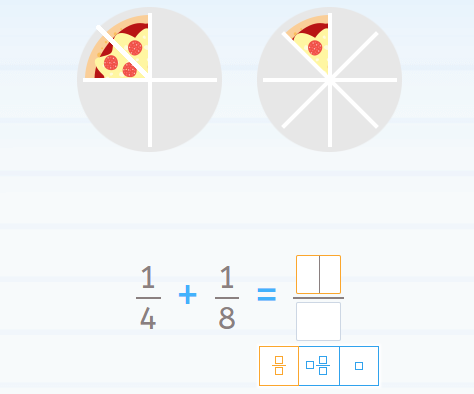
Well to start adding fractions with different denominators, you just need to remember some very simple relationships that you have probably heard of before.
We know that:
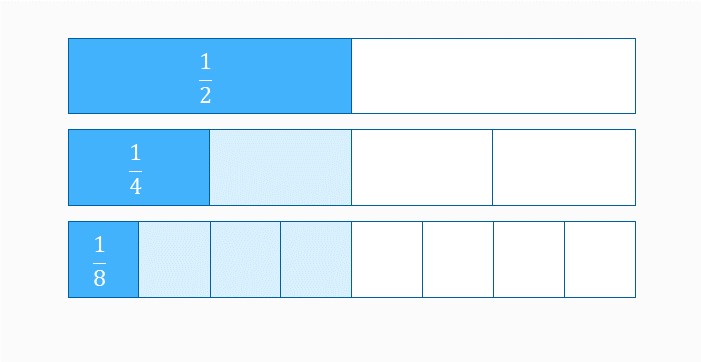
- One half is double one fourth and one fourth is double one eighth.
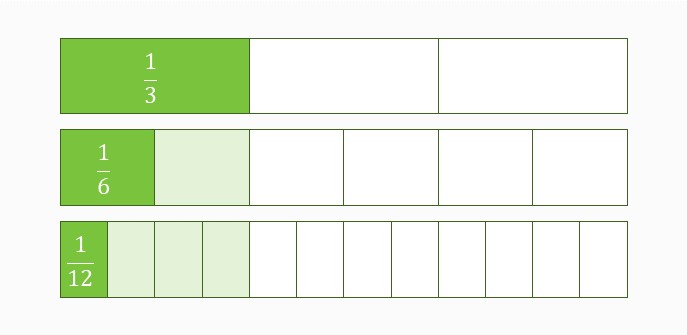
- One third is double one sixth.
- One fifth is double one tenth.
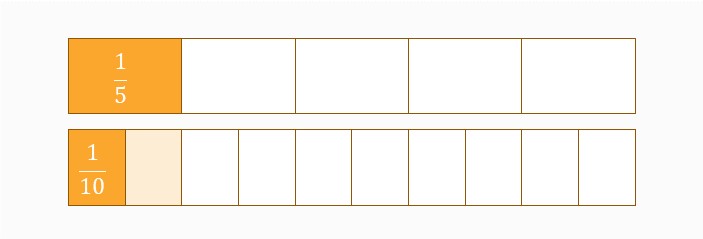
With this in mind, now you are ready to keep learning about adding fractions and much more. You can practice this concept and many more by registering for free with Smartick.
Learn More:
- Using Lego Blocks to Help with Addition of Fractions
- Practice Adding Fractions with Examples
- Learn about Fractions: Halves, Thirds and Fourths
- Homogeneous and Heterogeneous Fractions
- How to Add 3 Fractions with Different Denominators
A multidisciplinary and multicultural team made up of mathematicians, teachers, professors and other education professionals!
They strive to create the best math content possible.
- Mathematical Formulas: What Are They, How Are They Made and Types of Formulas? - 11/29/2024
- The Language of Functions and Graphs - 07/01/2024
- Educational Technology: The Christodoulou Test - 05/06/2024