Multiplication and division are closely related, given that division is the inverse operation of multiplication. When we divide, we look to separate into equal groups, while multiplication involves joining equal groups.
In today’s post, we’re going to learn to use multiplication as a strategy to solve division problems, which will be really useful for daily life!
We’ll start with a basic multiplication. If we have 4 x 5 = 20, its inverse relations (in the form of a division) will be the following:
20 ÷ 5 = 4
20 ÷ 4 = 5
In the same way, if we take the division 30 ÷ 3 = 10, its inverse relations (in the form of a multiplication) will be the following:
3 x 10 = 30
10 x 3 = 30
In both examples, we can see that we use the same three numbers. This is because when we multiply two numbers (which we call factors), we get a result that we call a product. If we divide this product by one of the factors, we get the other factor as a result.
Example of division solved through multiplication
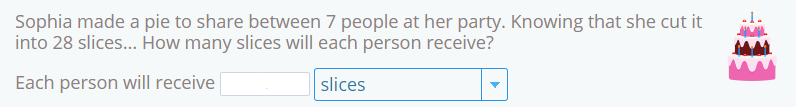
Here we have:
- A total number of objects: 28 slices in total
- A number of sets: 7 people
- Representation: 42 ÷ 7 = ___
To calculate the exact number of portions that will be given to each person, we have to look for a number that when multiplied by 7 gives us 28. What will it be?
7 x 1 = 7 7 x 6 = 42
7 x 2 = 14 7 x 7 = 49
7 x 3 = 21 7 x 8 = 56
7 x 4 = 28 7 x 9 = 63
7 x 5 = 35 7 x 10 = 70
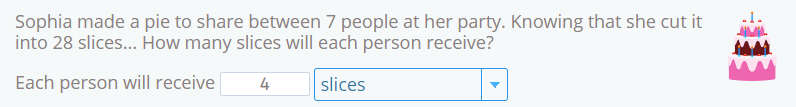
Great! 4 is the number that gives us 28 when we multiply it by 7. Since the multiplication is the inverse operation to division, 28 divided by 7 equals 4.
Therefore the answer to our exercise is:
Remember that if you want to improve your multiplication and division, the best thing to do is review the times tables and practice with our exercises. Either way, review our post on divisions and practice with our division exercises.
If you want to keep learning much more primary math, log in to Smartick and try it for free.
Learn More:
- What is Multiplication: Steps to Learn and Understand Multiplication
- Understanding the Division of Fractions
- Properties of Multiplication
- Practice Dividing with and without Remainders
- Division Exercises: Learning the Concept of Division
A multidisciplinary and multicultural team made up of mathematicians, teachers, professors and other education professionals!
They strive to create the best math content possible.
- The Language of Functions and Graphs - 07/01/2024
- Educational Technology: The Christodoulou Test - 05/06/2024
- Multiplication Activities in Smartick - 04/09/2024
I like it
This shows a very good direction.
For centuries mankind is choosing an improper word for the operation performed by /, : or ÷. What we call division is not the inverse of multiplication. A “division” always describes a relation between two numbers. What is the relation between 1 and 3? 0.3333…
Meaning that if you multiply 0.3333… by 3 you get 1 part and if you split 1 by 0,3333… you get 3 parts. Actual division (splitting) and multiplication are closely connected to the relation, but they are three sepparate things. In order to multiply something to get a value, you need to know what to multiply by, which is the relation. As well as you need to know what to split by to get a certain value, which again, is the relation between the two initial values. Splitting and multiplication are counterparts, but what we call division, what i call relation, is neither a multiplication or a splitting operation. That is what makes the concept of division so hard to grasp for many. A relation is its own sepparate value, but the wording of division does not distinguish between splitting and relating two values.
It is good but add more detail -_-