Is 2 A Prime Number?
Prime vs. Composite Numbers
Greetings, young math enthusiasts! Today, let’s set sail on a mathematical voyage to determine if 2 is a prime number, and learn more about the difference between prime and composite numbers.

What are Regular Prime Numbers?
Ever wondered what makes some numbers extra special? Meet regular primes, the stars of the math world! Let’s dive into their fun and quirky story!


No credit card required

No credit card required
What is a Regular Prime?
What are Prime and Composite Numbers?
What is an Irregular Prime?
What is the History Behind Regular Primes?
So, Are All Prime Numbers Regular?
What is the Smallest Regular Prime?
List of First 100 Regular Prime Numbers
What Other Kinds of Prime Numbers Are There?
What is a Regular Prime?
Definition
A regular prime is a prime number that meets specific mathematical criteria related to certain algebraic properties. These criteria involve the prime number’s behavior in relation to Bernoulli numbers and the class number of a cyclotomic field. Let’s break down these concepts to understand what makes a prime number regular.
Class Number Criterion
To understand the class number criterion, we need to explore some concepts in number theory:
Cyclotomic Field: This is a special type of number field. Imagine you have a circle, and you divide it into equal parts, like slicing a pizza. Each point where you make a cut corresponds to a complex number called a “root of unity.” These roots of unity generate a cyclotomic field.
Class Number: This measures the complexity of the cyclotomic field. It tells us about the number of distinct ways we can factorize numbers in this field. In simpler terms, it’s like counting the different paths in a complex network.
Now, for a prime number p to be considered regular, the class number of the cyclotomic field generated by p must not be divisible by p. This means that when you calculate the class number for this field, p shouldn’t fit evenly into it. If p doesn’t divide the class number, the prime is labeled as regular.
Kummer’s Criterion
Kummer’s criterion involves another set of special numbers called Bernoulli numbers:
- Bernoulli Numbers: These are a sequence of numbers that appear in many areas of mathematics, including number theory and calculus. They are named after the mathematician Jacob Bernoulli. These numbers are rational and are part of the formula for calculating sums of powers of integers.
To use Kummer’s criterion, we look at the first (p−3)/2 Bernoulli numbers, where p is the prime number we are testing. For example, if p is 5, we look at the first (5−3)/2=1 Bernoulli number. If p does not divide the numerator of any of these Bernoulli numbers, then p is considered a regular prime.
Let’s illustrate with an example:
- Suppose we want to test if 5 is a regular prime.
- The first Bernoulli number is B1=1/6
- We check if 5 divides the numerator of 1/6. Since 5 does not divide 1, 5 is regular according to Kummer’s criterion.
In summary, a prime number is regular if:
- It does not divide the class number of the cyclotomic field generated by that prime.
- It does not divide the numerator of any of the first (p−3)/2 Bernoulli numbers.
These criteria help mathematicians classify prime numbers and understand their properties in deeper algebraic structures. Regular primes behave in a predictable manner within these structures, making them a key topic in number theory.
What are Prime and Composite Numbers?
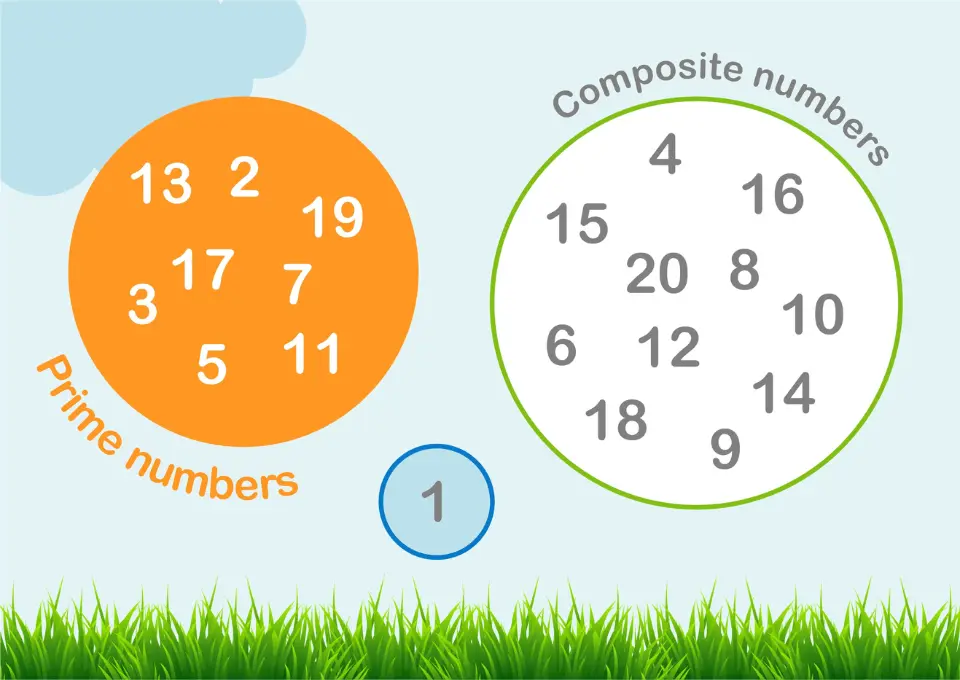
Prime Numbers
Prime numbers are the building blocks of mathematics. They are numbers greater than 1 that can only be divided evenly by 1 and themselves. For example, 2, 3, 5, and 7 are prime numbers. You can’t divide them evenly by any other numbers except for 1 and the number itself.
Composite Numbers
On the other hand, composite numbers are numbers that can be divided evenly by numbers other than 1 and themselves. For example, 4, 6, 8, and 9 are composite numbers because you can divide them by other numbers (like 2 or 3) and still get a whole number.
What is an Irregular Prime?
An irregular prime is a prime number that doesn’t follow the rules for regular primes. In other words, it fails the class number criterion or Kummer’s criterion. Irregular primes don’t fit neatly into the equations and properties that regular primes do. This makes them interesting and somewhat unpredictable in the world of mathematics.
What is the History Behind Regular Primes?
Kummer’s Discovery
The story of regular primes goes back to the mid-1800s. In 1850, Ernst Kummer made a significant discovery. He proved that a famous math problem called Fermat’s Last Theorem is true for prime numbers if they are regular. This was a huge deal because Fermat’s Last Theorem was a puzzle that stumped mathematicians for a long time. Kummer’s work showed that for primes that were regular, the theorem held true, which was a major breakthrough.
Genocchi’s Contribution
In 1852, another mathematician named Angelo Genocchi made an important discovery. He found that the first part of Fermat’s Last Theorem holds true if a prime number and a number three less than it are not both irregular. This was like finding another piece of the puzzle and added to the understanding of how primes behaved in these equations.
More Progress
Kummer continued his work in 1857, showing that to prove the first part of Fermat’s Last Theorem, it was enough to check if either a prime number and a number three less than it, or a prime number and a number five less than it, are not both irregular. This further refined the conditions under which the theorem could be proven.
Finding Irregular Primes
Kummer also worked on finding irregular primes. He listed all the irregular primes less than 165. Later, in 1963, mathematician D.H. Lehmer extended this list up to 10,000. In 1964, Selfridge and Pollack announced they had found irregular primes up to 25,000. Although their results weren’t published, Johnson confirmed that there was only one irregular pair for primes less than 30,000. More irregular primes were found in 1993, with the next irregular pair appearing much later.
So, Are All Prime Numbers Regular?
No, not all prime numbers are regular. Some are regular, and some are irregular. The irregular primes don’t follow the special rules that regular primes do. It’s like having two different groups within the world of prime numbers.
What is the Smallest Regular Prime?
The smallest regular prime is 2. This little number is special because it passes all the tests to be a regular prime. It’s like the baby of the prime number family, yet it holds a lot of power.
What is the Smallest Irregular Prime?
The smallest irregular prime is 37. Unlike the well-behaved regular primes, 37 breaks some of the mathematical rules and behaves unpredictably. It’s like the troublemaker of the prime number world.
List of First 100 Regular Prime Numbers

Here are the first 100 regular prime numbers:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97, 101, 103, 107, 109, 113, 127, 131, 137, 139, 149, 151, 157, 163, 167, 173, 179, 181, 191, 193, 197, 199, 211, 223, 227, 229, 233, 239, 241, 251, 257, 263, 269, 271, 277, 281, 283, 293, 307, 311, 313, 317, 331, 337, 347, 349, 353, 359, 367, 373, 379, 383, 389, 397, 401, 409, 419, 421, 431, 433, 439, 443, 449, 457, 461, 463, 467, 479, 487, 491, 499, 503, 509, 521, 523, 541.
And there you have it! Regular primes are a fascinating topic in the world of math. They show us how even numbers can have special properties and secrets waiting to be discovered. Who knows, maybe one day you’ll find a new kind of prime number!
What Other Kinds of Prime Numbers Are There?
In addition to sexy primes, there are many other interesting types of prime numbers. Here are a few:
Twin Primes: Twin primes are pairs of prime numbers that differ by two. Examples include (3, 5), (11, 13), and (17, 19). Twin primes are very similar to sexy primes but with a smaller difference.
Cousin Primes: Cousin primes are pairs of prime numbers that differ by four. Examples include (3, 7), (7, 11), and (19, 23). The difference of four gives them their unique name.
Mersenne Primes: Mersenne primes are prime numbers that are one less than a power of two. For example, 3 and 31 are Mersenne primes. These primes are named after the French mathematician Marin Mersenne.
Fermat Primes: Fermat primes are prime numbers of a special form involving powers of two. For example, 3 and 5 are Fermat primes. They are named after the French mathematician Pierre de Fermat.
Sexy Primes: Sexy primes are pairs of prime numbers that differ by six. Examples include (5, 11), (7, 13), and (11, 17). The term “sexy” comes from the Latin word for six, making these primes easy to remember.
Lucky Primes: Lucky primes are lucky numbers that are also prime. For example, 7 and 13 are lucky primes. Lucky numbers are a special sequence of integers generated by a particular sieving process similar to the Sieve of Eratosthenes.
Gaussian Primes: Gaussian primes are a type of prime number in the complex number system. An example is 3, which is prime in both the regular and complex number systems.
Happy Primes: Happy primes are prime numbers that are also happy numbers. A happy number is defined by a process where you repeatedly sum the squares of its digits until you reach 1. For example, 7 is a happy prime because it eventually reaches 1 through this process.
Learn More About Prime Numbers
© 2024 Smartick. All Rights Reserved.
