Is 2 A Prime Number?

Prime vs. Composite Numbers

Greetings, young math enthusiasts! Today, let’s set sail on a mathematical voyage to determine if 2 is a prime number, and learn more about the difference between prime and composite numbers.

What are Sophie Germain Prime Numbers?

An educational illustration titled ‘Sophie Germain Prime Numbers’ featuring two playful cartoon characters with glasses and lab coats standing in grass under a wooden sign.

What are Safe and Sophie Germain Primes?

What are Prime and Composite Numbers?

What are Sophie Germain Primes Used For?

What is the History Behind Sophie Germain Primes?

Are There Infinite Sophie Germain Primes?

What is the Largest Sophie Germain Prime?

Is 53 a Sophie Germain Prime?

Is 27 a Sophie Germain Prime?

Is 47 a Germain Prime?

Is 2 a Germain Prime?

List of First 100 Sophie Germain Primes

What Other Kinds of Prime Numbers Are There?

What are Safe and Sophie Germain Primes?

Sophie Germain Primes

A Sophie Germain prime is a prime number $p$ such that $2 p + 1$ is also prime. For example, 5 is a Sophie Germain prime because $\times 5 + 1 = 11$ , and 11 is also a prime number. These primes are named after the French mathematician Sophie Germain, who made significant contributions to number theory.

Safe Primes

A safe prime is a prime number of the form $2 p + 1$ , where $p$ is also a prime number. In other words, if $p$ is a Sophie Germain prime, then $2 p + 1$ is a safe prime. For example, since 5 is a Sophie Germain prime, 11 is a safe prime.

What are Prime and Composite Numbers?

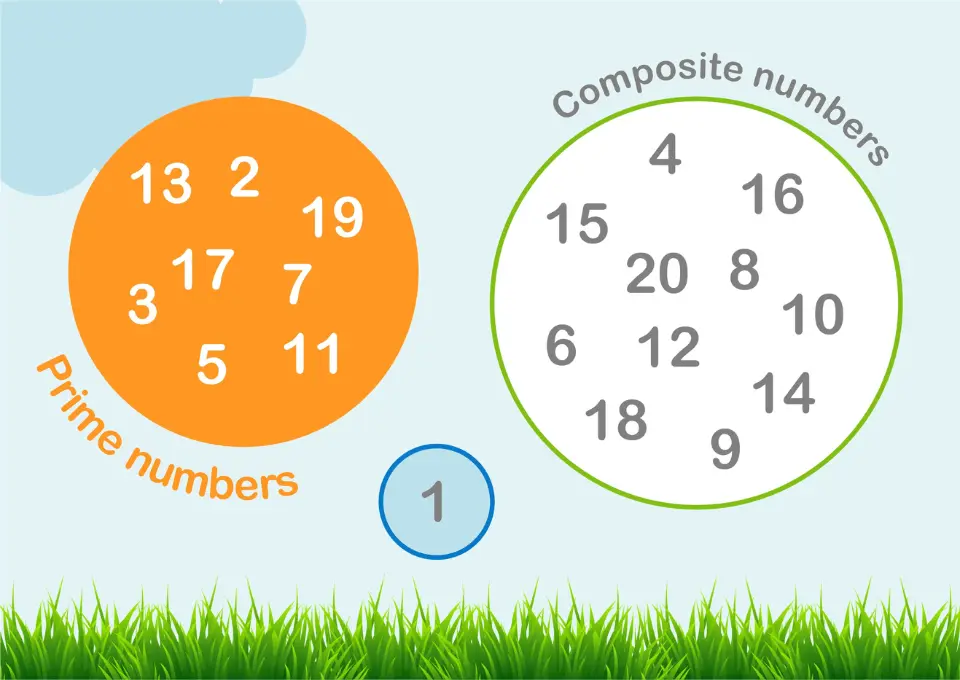
Prime Numbers

Prime numbers are like the building blocks of mathematics. They are numbers greater than 1 that can only be divided evenly by 1 and themselves. For example, 2, 3, 5, and 7 are prime numbers. You can’t divide them evenly by any other numbers except for 1 and the number itself.

Composite Numbers

On the other hand, composite numbers are numbers that can be divided evenly by numbers other than 1 and themselves. For example, 4, 6, 8, and 9 are composite numbers because you can divide them by other numbers (like 2 or 3) and still get a whole number.

What are Sophie Germain Primes Used For?

Sophie Germain primes are used in various fields of mathematics and cryptography. In cryptography, they are particularly valuable for creating secure keys for encryption. This is because the properties of Sophie Germain primes make it difficult for anyone to break the encryption without knowing the key.

These primes are also studied in number theory for their unique properties and relationships with other prime numbers. They help mathematicians understand the distribution and patterns of primes, which is a fundamental aspect of number theory.

Are There Infinite Sophie Germain Primes?

Mathematicians believe that there are infinitely many Sophie Germain primes, but this has not been proven. This is similar to the case with prime numbers themselves; while we know there are infinitely many primes, the same cannot yet be definitively said for Sophie Germain primes. The search for more Sophie Germain primes continues, and each new discovery helps us understand more about their distribution.

What is the Largest Sophie Germain Prime?

The largest known Sophie Germain prime changes as mathematicians and computer scientists discover new ones. As of now, the largest known Sophie Germain prime has hundreds of thousands of digits. These massive primes are discovered using advanced computer algorithms and are a testament to the power of modern computational techniques.

Is 53 a Sophie Germain Prime?

To determine if 53 is a Sophie Germain prime, we need to check if is also prime.

Since 107 is a prime number, 53 is indeed a Sophie Germain prime.

Is 27 a Sophie Germain Prime?

To see if 27 is a Sophie Germain prime, we check if .

Since 55 is not a prime number (it can be divided by 5 and 11), 27 is not a Sophie Germain prime.

Is 47 a Germain Prime?

To determine if 47 is a Sophie Germain prime, we check if is also prime.

Since 95 is not a prime number (it can be divided by 5 and 19), 47 is not a Sophie Germain prime.

Is 2 a Germain Prime?

Let’s check if 2 is a Sophie Germain prime by seeing if is also prime.

Since 5 is a prime number, 2 is indeed a Sophie Germain prime.

List of First 100 Sophie Germain Primes

Here are the first 100 Sophie Germain primes:

2, 3, 5, 11, 23, 29, 41, 53, 83, 89, 113, 131, 173, 179, 191, 233, 239, 269, 281, 293, 311, 359, 419, 431, 443, 491, 509, 593, 641, 653, 659, 719, 761, 809, 821, 863, 881, 953, 1013, 1049, 1103, 1223, 1259, 1277, 1283, 1409, 1433, 1439, 1451, 1493, 1523, 1613, 1709, 1733, 1753, 1823, 1949, 1993, 2003, 2063, 2129, 2153, 2203, 2333, 2383, 2441, 2459, 2579, 2693, 2729, 2789, 2903, 2963, 2999, 3203, 3299, 3329, 3359, 3449, 3463, 3491, 3539, 3623, 3779, 3823, 3889, 3919, 4013, 4049, 4073, 4091, 4133, 4229, 4259, 4349, 4409, 4483, 4513, 4547, 4639, 4673.

These Sophie Germain primes have fascinating properties and play a crucial role in both theoretical and applied mathematics. They help us understand more about the patterns and behaviors of prime numbers, making them a key topic of study in number theory. So, the next time you encounter a prime number, think about whether it might be a Sophie Germain prime and the interesting history and properties it carries with it!

What Other Kinds of Prime Numbers Are There?

In addition to sexy primes, there are many other interesting types of prime numbers. Here are a few:

Twin Primes: Twin primes are pairs of prime numbers that differ by two. Examples include (3, 5), (11, 13), and (17, 19). Twin primes are very similar to sexy primes but with a smaller difference.
Cousin Primes: Cousin primes are pairs of prime numbers that differ by four. Examples include (3, 7), (7, 11), and (19, 23). The difference of four gives them their unique name.
Mersenne Primes: Mersenne primes are prime numbers that are one less than a power of two. For example, 3 and 31 are Mersenne primes. These primes are named after the French mathematician Marin Mersenne.
Fermat Primes: Fermat primes are prime numbers of a special form involving powers of two. For example, 3 and 5 are Fermat primes. They are named after the French mathematician Pierre de Fermat.
Sexy Primes: Sexy primes are pairs of prime numbers that differ by six. Examples include (5, 11), (7, 13), and (11, 17). The term “sexy” comes from the Latin word for six, making these primes easy to remember.
Lucky Primes: Lucky primes are lucky numbers that are also prime. For example, 7 and 13 are lucky primes. Lucky numbers are a special sequence of integers generated by a particular sieving process similar to the Sieve of Eratosthenes.
Gaussian Primes: Gaussian primes are a type of prime number in the complex number system. An example is 3, which is prime in both the regular and complex number systems.
Happy Primes: Happy primes are prime numbers that are also happy numbers. A happy number is defined by a process where you repeatedly sum the squares of its digits until you reach 1. For example, 7 is a happy prime because it eventually reaches 1 through this process.