Is 2 A Prime Number?

Prime vs. Composite Numbers

Greetings, young math enthusiasts! Today, let’s set sail on a mathematical voyage to determine if 2 is a prime number, and learn more about the difference between prime and composite numbers.

What are Twin Prime Numbers? - All You Should Know!

Twin prime numbers are like, well… twins! in the number world they always appear side by side with just one number between them! These pairs of primes bring an extra spark of joy to the study of mathematics, making numbers even more fascinating.

What are Twin Prime Numbers?

What are Prime and Composite Numbers?

How Many Twin Primes Exist?

What is the Smallest Twin Prime?

What is the Twin Prime Problem?

How Rare Are Twin Primes?

Are Twin Primes Infinite?

What is the Significance of Twin Prime Numbers?

Do Triplet Primes Exist?

What are the Twin Primes of 24?

Additional Key Concepts

What are Twin Prime Numbers?

Twin prime numbers are special pairs of prime numbers that are only two numbers apart. For example, 3 and 5 are twin primes because they are both prime numbers, and the difference between them is 2.

Twin primes are like special buddies in the world of numbers. They always stay close together.

A twin prime is a prime number that has another prime number exactly two numbers away from it, forming a pair of twin prime pairs with a difference of 2.

Basics First, What are Prime and Composite Numbers?

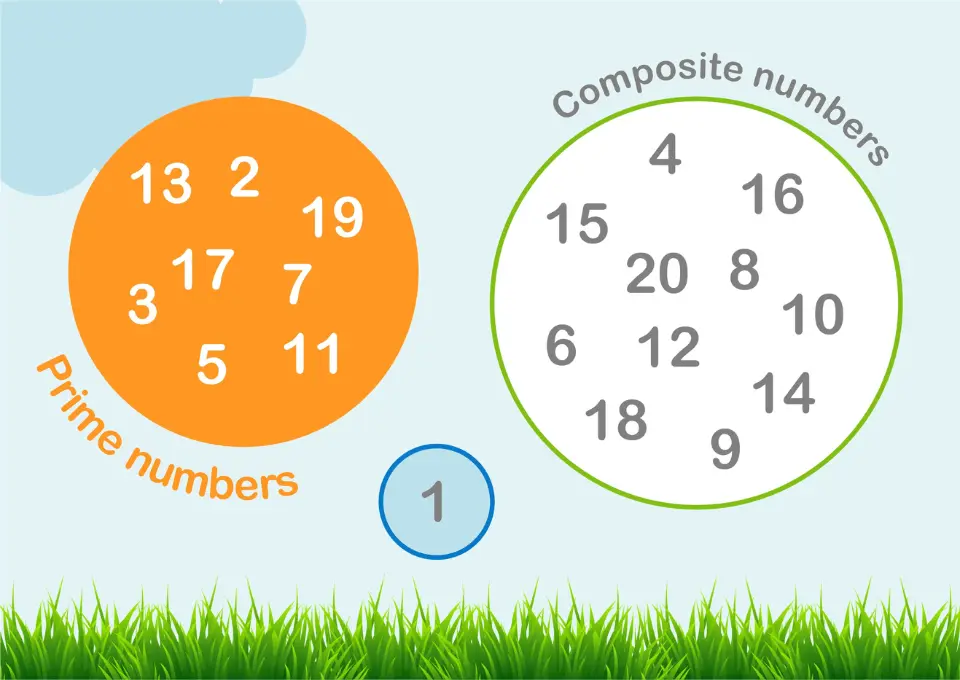To understand twin prime numbers, we first need to know what prime and composite numbers are.

Prime Numbers: A prime number is a number greater than 1 that has no divisors other than 1 and itself. In other words, it can only be divided by 1 and the number itself without leaving a remainder. Some examples of prime numbers are 2, 3, 5, 7, 11, and 13. 17 is also a prime number! Primes become less frequent as numbers get larger, but they are still found throughout the number line.

Composite Numbers: A composite number is a number greater than 1 that has more than two divisors. This means it can be divided by 1, itself, and at least one other number. For example, 4 is a composite number because it can be divided by 1, 2, and 4. Other examples of composite numbers are 6, 8, 9, 10, and 12.

How Many Twin Primes Exist?

The exact number of twin primes is unknown. Mathematicians have discovered many twin primes, but they haven’t found all of them because the numbers go on forever.

However, they believe that there are infinitely many twin primes. This means there are endless pairs of two twin primes handing together, even though we don’t know all of them yet. The twin prime conjecture states that there are infinitely many pairs of prime numbers that differ by 2.

In 2013, an American mathematician named Zhang Yitang made an important discovery. He proved that there are an infinite number of pairs of prime numbers separated by a fixed distance that is greater than 2 but less than 70 million. This means that there are endless pairs of prime numbers, where the difference between them is more than 2 but less than or equal to 70 million.

Later, another mathematician named James Maynard improved Zhang’s result. He showed that this fixed distance could be reduced to 600. Then, in 2014, a team of scientists led by Terence Tao, working on the Polymath Project, improved this even further to 246.

The goal of these discoveries is to show that there are infinitely many twin primes.

What is the Smallest Twin Prime?

The smallest twin prime is the pair 3 and 5. These two numbers are both prime, and the difference between them is 2. Another pair of twin primes is 5 and 7. As we look at more numbers, we find more twin primes, but 3 and 5 are the smallest.

What is the Twin Prime Problem?

The twin prime problem is a famous question in mathematics. It asks whether there are infinitely many twin primes. Even though mathematicians have found many twin primes and believe there are infinitely many, they have not yet been able to prove it for sure. This problem has puzzled mathematicians for a long time and is still an unsolved mystery in the world of number theory.

How Rare Are Twin Primes?

As numbers get larger, twin primes become rarer.

In the smaller numbers, you can find many pairs of twin primes, but as you count higher and higher, they become less common. For example, in the numbers from 1 to 100, there are eight pairs of twin primes. However, if you look at the numbers from 1 to 1,000,000, there are only 8,169 pairs of twin primes.

So, while there are still many twin primes, they are less frequent as the numbers get bigger. This is related to the prime gap, which is the difference between consecutive primes. As numbers get larger, these gaps between primes can vary widely.

Are Twin Primes Infinite?

As we already stated, most mathematicians believe that there are infinitely many twin primes, but this hasn’t been proven yet. The twin prime conjecture is a hypothesis that suggests there are endless pairs of twin primes. Even though this has not been confirmed, the evidence so far supports this idea.

Mathematicians continue to search for proof that will show, once and for all, that twin primes are indeed infinite. This conjecture is sometimes called Euclid’s twin prime conjecture, named after the famous ancient Greek mathematician Euclid, who studied prime numbers extensively.

What is the Significance of Twin Prime Numbers?

Twin prime numbers are important in mathematics because they help mathematicians understand the distribution of prime numbers.

Prime numbers are the building blocks of all other numbers, and studying twin primes gives insight into how primes are spaced out among the other numbers. This knowledge can be useful in various fields, including computer science and cryptography, where prime numbers play a key role in securing information. The properties of twin primes are a subject of deep interest in number theory and have led to many interesting discoveries.

Do Triplet Primes Exist?

What are Prime Triplets?

Prime triplets are groups of three prime numbers that are closely spaced together. Just like twin primes are pairs of prime numbers that differ by only 2, prime triplets are sets of three prime numbers that have specific differences between them.

3, 5, and 7: A Unique Prime Triplet

The numbers 3, 5, and 7 form a unique prime triplet. These three numbers are prime, and the differences between them are 2. This set is special because it is the only group of three consecutive prime numbers where each number in the set is prime. For example, 3 is a prime number, 5 is a prime number, and 7 is a prime number. The differences between these numbers (5 – 3 = 2 and 7 – 5 = 2) make them a special group known as a prime triplet.

Infinite Prime Triplets?

Many sources on the internet say that there are no other sets of three consecutive prime numbers like 3, 5, and 7. However, there is a conjecture about prime triplets that suggests there might be infinitely many prime triplets. This conjecture states that for any given set of three prime numbers (p, p+2, and p+6 or p, p+4, and p+6), there might be an infinite number of such sets.

According to the Wikipedia page on prime triplets, this idea is based on the patterns observed in the distribution of prime numbers. Even though mathematicians haven’t proven it yet, some believe that there could be infinitely many prime triplets following certain patterns.

What are the Twin Primes of 24?

When we talk about twin primes of 24, we are looking for pairs of twin primes that surround the number 24. The twin primes near 24 are 23 and 25. However, 25 is not a prime number, so there are no twin primes directly around 24. Instead, we look at the closest twin primes, which are 29 and 31. These two numbers are both prime, and they differ by 2, making them twin primes.

Additional Key Concepts

Prime Number Theorem

The prime number theorem describes the asymptotic distribution of prime numbers among the positive integers. It provides a general understanding of how primes are spaced, even though it does not specifically address twin primes.

Polignac’s Conjecture

Polignac’s conjecture is another hypothesis in number theory, proposed by Alphonse de Polignac in 1846. It generalizes the twin prime conjecture by suggesting that for any even number $k$ , there are infinitely many pairs of prime numbers that differ by .

Brun’s Theorem

Brun’s theorem, proved by Viggo Brun in 1919, states that the sum of the reciprocals of the twin primes converges. This is an important result in number theory because it contrasts with the sum of the reciprocals of all prime numbers, which diverges.

Sieve Methods

Sieve methods are a set of techniques used in number theory to count, or more generally, describe the structure of prime numbers and related sets of numbers. The sieve of Eratosthenes is a famous example used to find all prime numbers up to a certain limit.

Notable Mathematicians

Terence Tao: A renowned mathematician who has made significant contributions to number theory, including work on prime gaps.
Yitang Zhang: Known for his work on bounded gaps between primes, which brought new insights into the distribution of prime numbers.
James Maynard: A mathematician who has made breakthroughs in understanding small gaps between primes.

These concepts and mathematicians have all played a role in advancing our understanding of twin primes and prime numbers in general.

Before you number away…

Twin prime numbers are fascinating pairs of prime numbers that are only two numbers apart. To understand them better, we first learned about prime and composite numbers. Even though we know many twin primes, the exact number is still unknown, and mathematicians believe there might be infinitely many of them. The smallest twin prime is 3 and 5, and the twin prime problem is an important unsolved question in mathematics. While twin primes become rarer as numbers get larger, their study helps mathematicians understand the distribution of prime numbers. Twin primes hold a special place in the world of math and continue to intrigue and challenge mathematicians everywhere.