Is 2 A Prime Number?
Prime vs. Composite Numbers
Greetings, young math enthusiasts! Today, let’s set sail on a mathematical voyage to determine if 2 is a prime number, and learn more about the difference between prime and composite numbers.

What are Wilson Prime Numbers?
Imagine finding a prime number with a secret mathematical twist! Welcome to the world of Wilson primes, where numbers play by unique rules. Jump in!


No credit card required

No credit card required
What is a Wilson Prime?
What are Prime and Composite Numbers?
What is the Wilson’s Prime Algorithm?
What is the Wilson’s Little Theorem?
How Many Wilson Prime Numbers Exist?
What is the Smallest Wilson Prime?
Can We Find the Fourth Wilson Prime?
What Other Kinds of Prime Numbers Are There?
What is an Wilson Prime?
Wilson primes are a unique type of prime number with special properties related to factorials. A prime number ppp is considered a Wilson prime if it satisfies the condition: p2 divides (p−1)! + 1. This mathematical relationship makes Wilson primes rare and intriguing for mathematicians.
What are Prime and Composite Numbers?
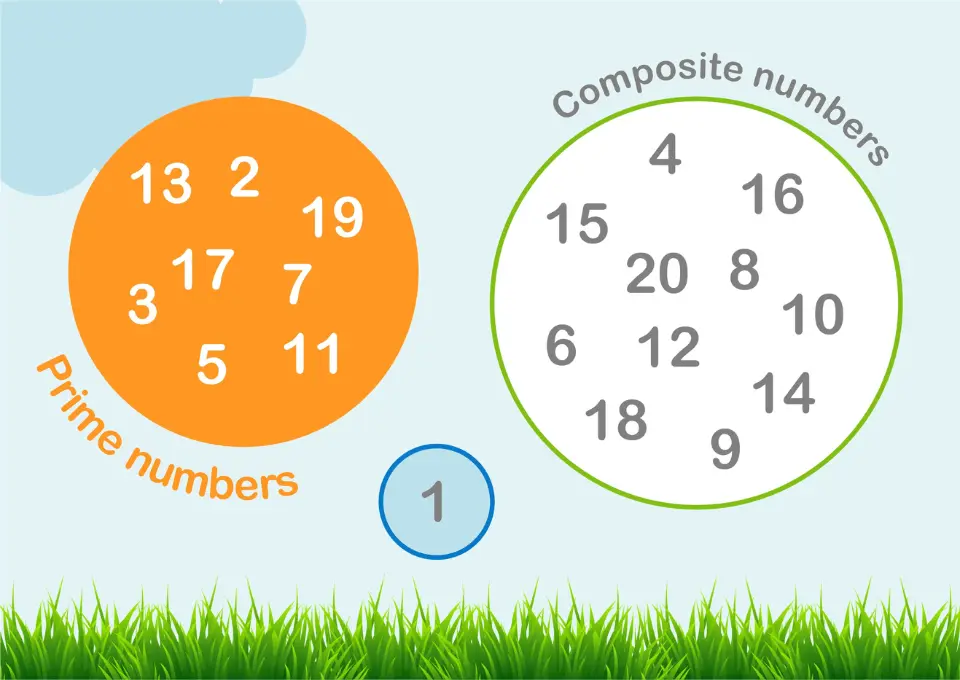
Prime numbers are numbers greater than 1 that have no divisors other than 1 and themselves. Examples of prime numbers include 2, 3, 5, and 7. On the other hand, composite numbers are numbers greater than 1 that have more than two divisors.
Examples of composite numbers are 4, 6, 8, and 9. Understanding the difference between prime and composite numbers is fundamental in number theory.
What is the Wilson’s Prime Algorithm?
The Wilson’s prime algorithm is a method to determine whether a given prime number p is a Wilson prime. According to the algorithm, you compute (p−1)!+1 and check if it is divisible by p2. If the result is an integer, then ppp is a Wilson prime. This algorithm helps identify Wilson primes but is computationally intensive due to the rapid growth of factorials.
What is the Wilson’s Little Theorem?
Wilson’s Theorem is a fundamental result in number theory that states: for any prime number p, (p−1)! = −1 (mod p). This theorem implies that the factorial of one less than a prime number, when divided by the prime, leaves a remainder of -1. Wilson primes extend this theorem by involving the square of the prime number in the divisibility condition.
How Many Wilson Prime Numbers Exist?
Currently, only three Wilson primes are known: 5, 13, and 563. These primes are the only ones that satisfy the Wilson prime condition. Despite extensive computational searches, no additional Wilson primes have been discovered, highlighting their rarity and special nature.
What is the Smallest Wilson Prime?
The smallest Wilson prime is 5. It satisfies the condition as (5−1)! + 1 = 24 + 1=25, and 25 is divisible by 525^252. This makes 5 the smallest and one of the rare Wilson primes known.
Can We Find the Fourth Wilson Prime?
Finding the fourth Wilson prime is a significant challenge for mathematicians. The difficulty lies in the computational complexity of calculating large factorials and verifying the divisibility condition. While extensive searches have been conducted, no new Wilson primes have been found beyond the known three. The quest to discover more Wilson primes continues to intrigue researchers.
What Other Kinds of Prime Numbers Are There?
In addition to sexy primes, there are many other interesting types of prime numbers. Here are a few:
-
Twin Primes: Twin primes are pairs of prime numbers that differ by two. Examples include (3, 5), (11, 13), and (17, 19). Twin primes are very similar to sexy primes but with a smaller difference.
-
Cousin Primes: Cousin primes are pairs of prime numbers that differ by four. Examples include (3, 7), (7, 11), and (19, 23). The difference of four gives them their unique name.
-
Mersenne Primes: Mersenne primes are prime numbers that are one less than a power of two. For example, 3 and 31 are Mersenne primes. These primes are named after the French mathematician Marin Mersenne.
-
Fermat Primes: Fermat primes are prime numbers of a special form involving powers of two. For example, 3 and 5 are Fermat primes. They are named after the French mathematician Pierre de Fermat.
-
Sexy Primes: Sexy primes are pairs of prime numbers that differ by six. Examples include (5, 11), (7, 13), and (11, 17). The term “sexy” comes from the Latin word for six, making these primes easy to remember.
-
Lucky Primes: Lucky primes are lucky numbers that are also prime. For example, 7 and 13 are lucky primes. Lucky numbers are a special sequence of integers generated by a particular sieving process similar to the Sieve of Eratosthenes.
-
Gaussian Primes: Gaussian primes are a type of prime number in the complex number system. An example is 3, which is prime in both the regular and complex number systems.
-
Happy Primes: Happy primes are prime numbers that are also happy numbers. A happy number is defined by a process where you repeatedly sum the squares of its digits until you reach 1. For example, 7 is a happy prime because it eventually reaches 1 through this process.
Conclusion
Emirp prime numbers are an exciting and unique part of mathematics. They are prime numbers that remain prime even when their digits are reversed, making them extra special. Understanding prime and composite numbers helps us see why emirp primes are so interesting. With an infinite number of emirp primes, mathematicians have endless numbers to discover and study.
Alongside other fascinating types of prime numbers like sexy primes, twin primes, and Mersenne primes, emirp primes add to the rich and diverse world of number theory. By learning about these different kinds of prime numbers, we can appreciate the beauty and complexity of mathematics.
Learn More About Prime Numbers
© 2024 Smartick. All Rights Reserved.
